Support Topics
Support Topics / Engineer's Studio® Maintenance/Support
Differences among setting methods of residual displacement
  Overview
residual displacement verification is conducted according to the specifications for highway bridges, part V Seismic Design (2002-2012) and documents below:
  1. Case studies of seismic retrofitting method for existing bridges written by Japan Bridge Engineering Center, April 2005, p.ll-97
  2. Design examples and checkpoints for reinforced concrete pier, engineering science published by Sougou Doboku Kenkyusho. Co.,Ltd, June 2002.

The following are the features:
  1. Deformation quantity δpr is calculated by removing horizontal displacement due to the rotation of the foundation or that of foundation skeleton itself from maximum response displacement of pier top node. (Fig. 1)
    Fig. 1 Conceptual diagram of δpr
      δpr = δt + h1*θt - δb -h*θb
      δpr: deformation quantity of pier skeleton
      δt: horizontal displacement of the top of pier
      δb: horizontal displacement of the foundation basal plane
    *Described as displacement of the pier base in the document 1, and as displacement of the foundation basal plane in the document 2. Considered as the footing basal plane in Engineer's Studio®.
      θt : rotate angle of the pier top
      θb: rotate angle of the foundation basal plane
       h: height from the foundation basal plane to the position in the upper structure where inertia force acts
       h1: height from the top of pier to the position in the upper structure where inertia force acts
  2. In each step calculates residual displacement δR and does the max value among them. (Fig. 2)
    　　　δR = cR*(μr-1)(1-r) δy
    　　　μr = δpr / δy
    Fig. 2 Calculates the max value from the time history of δR.

  3. When receiving biaxial bending such as a curved bridge and a skew bridge, program automatically calculates the yield displacement in the direction of the maximum response displacement.
  4. Yield displacement δy is calculated by the method of obtaining coseismic horizontal strength in the specifications for highway bridges, part V Seismic Design (2002-2012). (*it is calculated exactly in our product "Pier design", but simply in Engineer's Studio® by the method of integrating the curvature distribution in the figure 3.)
    Fig. 3 Curvature distribution of RC pier
　Differences between the pier foundation and the foundation bottom
h in the figure 3 varies by presence or absence of the footing.

With footing
Enter the height from the foundation bottom in h and the footing thickness in h2 (Fig. 4).

Without footing
Enter the height from the pier foundation in h and 0 in h2 (Fig. 5).
Fig. 4 With footing Fig.5 Without footing
　Example
An input example for the pier like the figure 6 is below. It is 2.6 meters high from the top of the beam to the position in the upper structure where inertia force acts. This is the dynamic analysis which doesn't take the basic spring into account, consider the footing bottom to be fixed, and applies the type ll seismic movement 3 wave form to the direction of the bridge axis. It has the M-φ element in the post foundation and the elastic beam element in the middle part of the post.
Fig. 6 elliptical shaped pier (10 m high, 2 m thick in bottom)

Case 1
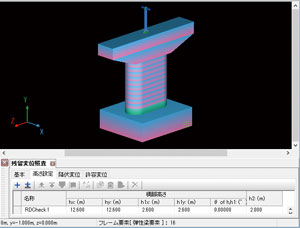
This is an example of the modeling of the footing and that of the position of the gravity center in the upper structure (Fig. 7). Mass 598t, which corresponds to the shared weight of the upper structure, is in 2.6m high above the top of the beam. The surround of the bridge axis of the spring element that means the bearing is free at right angles in order not to let the moment affect the top of the beam. In this case, the height would be entered as "h=12.6m, h1=2.6m, h2=2m". Yield displacement would be 41mm in 2.6m above the top of the beam.

Case 2
This is the modeling without the footing and the upper structure position like the figure 8. Mass 598t, which corresponds to the shared weight of the upper structure, is located at the top of the beam. The height would be entered as "h=8m, h1=0m, h2=0m", and the yield displacement would be 23mm at the top of the beam.

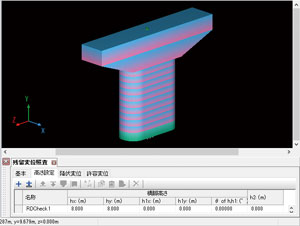Fig. 7 With the footing and the gravity center position
of the upper structure 		Fig. 8 Without the footing and the gravity center position
of the upper structure
　Result Comparizon
The results are shown in the figure 9 and 10. Both of the residual displacement δR and the allowable value δRa are larger by 30% in the case 1. Thus the ratio of the allowable value is almost the same (difference of about 3%).
Fig. 9 Result of case 1 Fig. 10 Result of case 2
　Conclusion
Case 1 is the model like taking out one from the entire system. In the case 2, the seismic horizontal strength method of the single post RC pier is modelized in regard to the direction of the bridge axis. By the modeling method, we can see that the difference in the residual displacement amount and the allowable value, but the design safe rate is almost the same. The residual displacement was 4.7mm in the static verification.
If exported from our product "Pier Design", the data of the skeleton model would be as that of the case 1 and the residual displacement as that of the case 2.
The result of the residual displacement verification would be largely similar to that of the case 2.
Since the exact modelization method is unclear as it is now, it would be grateful if these case studies about the modeling and the input of the residual displacement verification help your practical design.

Previous		 　　
Index
(Up&Coming 2016 Summer issue)
Back
Up&Coming
FORUM8